Instead of waiting for the realization of the state of nature, the principal can offer to the agent, at the ex ante stage (date t = 0), a contract that ensures ex post efficiency under some rather weak conditions, as we see in the following.
This contract can only be written in terms of the verifiable variables available to the trading partners, namely the transfer t and the production level q just as in chapter 2. For instance, a contract saying, “If state θ realizes, the agent must produce q∗(θ) and be paid t∗(θ) by the principal” cannot be enforced because the state of nature θ is not verifiable at the ex post contracting stage and consequently cannot be written into a contract. However, a nonlinear price t(q) or a menu ![]() is a feasible instrument at the ex ante stage.
is a feasible instrument at the ex ante stage.
When he accepts such a contract, the agent anticipates that his choice of outputs q(θ) in state θ will satisfy the following interim constraints:
![]()
for all q˜ in the domain of t(·) and all θ in Θ.
These constraints are the same as the standard incentive compatibility con-straints highlighted in chapter 2 as the reader will have recognized. Hence, there is a formal correspondence between the case where contracting takes place under asymmetric information between the principal and the agent, and the case of ex ante contracting when the state of nature is not verifiable. The revelation prin- ciple still applies in this context and the class of truthful direct revelation mech-anisms of the form ![]() is enough to describe all feasible contracts that command trade at date t = 2 and that can be signed at date t = 0 between the principal and the agent. Pushing this analogy, from now on we call these mecha- nisms incentive compatible contracts.
is enough to describe all feasible contracts that command trade at date t = 2 and that can be signed at date t = 0 between the principal and the agent. Pushing this analogy, from now on we call these mecha- nisms incentive compatible contracts.
The fact that the principal knows θ ex post is not used in such a mechanism. The benefit of incentive compatible contracts is that there is no need for the principal to act ex post (i.e., at date t = 2), as shown in figure 6.3. Only the agent reports the state of nature. The mechanism is thus not very demanding on the communication side and could be attractive if those communication costs were explicitly modeled.
We already know from section 2.11.1 that the first-best outcome can still be achieved with ex ante contracting, provided that the agent is risk neutral in a two- type environment. Nevertheless, note that the transfers with ex ante contracting are different from those obtained with no contract at date t = 0, as we can easily observe by comparing the results of sections 2.11.1 and 6.1. The reason for this difference is simple. With no contract at all, the transfers t∗ = θq∗ and t¯* = θ¯q¯*, which are offered by the principal at date t = 2, are no longer incentive compatible,3 as is requested with ex ante contracting.
However, this first-best implementation generalizes to several types and more general utility functions V = S(q, θ) − t for the principal and U = t − C(q, θ) for the agent if the Spence-Mirrlees properties Sqθ < 0 and Cqθ > 0 are both satis- fied. Otherwise, the second-order conditions for incentive compatibility may create some inefficiencies and may require some bunching, as in the case of nonrespon- siveness in section 2.10.2. When second-order conditions impose some pooling over several types, there may exist a real trade-off between an ex ante inefficient contract and an ex post negotiation leaving only a partial bargaining power to the principal.
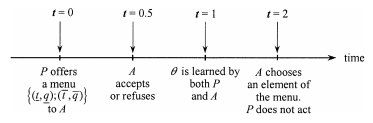
Figure 6.3: Timing with Ex Ante Contracting and Nonverifiability
An incentive compatible contract may also be useful if the principal is risk- averse and wants to obtain some insurance from the agent. Making the agent residual claimant for the gains from trade and reaping all of his profit with an up-front payment helps the principal to achieve the first-best outcome.4 However, as is also shown in section 2.11, ex ante contracting fails to achieve efficiency when the agent is risk-averse. The nonverifiability of the state of nature may then conflict with the insurance concern of the agent if the principal offers an incentive compatible contract. Therefore, section 2.11.2 also provides an analysis of the efficiency loss incurred when ex ante contracting limited to incentive compatible contracts takes place in a world of nonverifiability and risk aversion.
Green and Laffont (1992) characterized the incentive compatible contracts that optimize the principal’s expected welfare when ex post renegotiation led by the principal takes the utility levels achieved by those contracts as status quo utility payoffs.
Source: Laffont Jean-Jacques, Martimort David (2002), The Theory of Incentives: The Principal-Agent Model, Princeton University Press.
